Forum

Question about proof of theorem 13.4.25 in D & F (covered in lecture 3)

Question about proof of theorem 13.4.25 in D & F (covered in lecture 3)

by Benjamin Andersson -
Number of replies: 2
It seems like the induction in the proof below (only partially written up; note that this is not the full proof) is a bit more general than "just" over elements f(x) in F[x]:










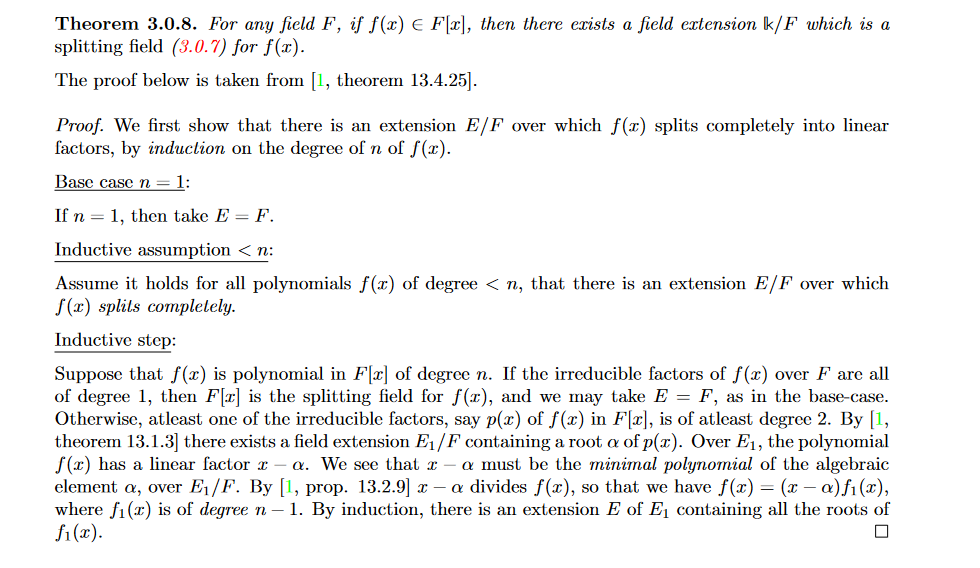
Am I correct that the induction-hypothesis should be something like: "Assume that for every field extension K/F, and every polynomial g(x) in K[x] of degree  < n (strong induction), there exists some extension field E/K such that g(x) splits completely into linear factors in E[x] (indeed, E/F if K/F and E/K)".

My formulation is motivated by the fact that f_1(x) is not neccessarily a polynomial in F[x]; so to apply the inductive assumption, we need something stronger than "every polynomial f(x) in F[x] of deg < n has a field extension E containing all the roots of f(x)".

P.s. I am not sure how you formulated this in class, I am not claiming you made a mistake, but I am not sure this was highlighted. Unless I am stupid and missing something obvious.

Do you agree?

Best,

Ben

In reply to Benjamin Andersson

Re: Question about proof of theorem 13.4.25 in D & F (covered in lecture 3)

by Sofia Tirabassi -

Hello,

Your induction  HP works, but also the one  stated above.

You  are right that f1 need not be a poly in F[x] (actually in the proof is never there). But you do not apply the induction HP to F and f1, you apply it to F(alpha) f1

Since in the HP they say for every field you can take  the field to be F(alpha). Then you get an extension E where f1 splits, but in the same extension F splits.


Actually I do nit think you even need strong induction since the degree is decreased just of 1...


I hope this clarifies


In reply to Sofia Tirabassi

Sv: Re: Question about proof of theorem 13.4.25 in D & F (covered in lecture 3)

by Benjamin Andersson -
I think I see what you are saying. The bit about doing induction over F(alpha) and f_1 clarified things! I am not sure D & F state it like this though (I don't have the book here).

And yes, you are right, as far as I can see, we don't need strong induction, f_1 is of degree n-1.

Best,

Ben